Poker combines skill and strategy with elements of probability, psychology, and game theory. To become a successful player, it is essential to have a solid understanding of the poker strategy math concepts that underpin the game.
Poker math is a set of principles and techniques that use mathematical concepts to help you make more informed decisions at the table. By understanding these concepts and using them to inform your decision-making process, you can improve your chances of winning in the long run.
The Basics Of Poker Math
The math in poker ranges from super simple calculations to incredibly complex GTO crunching. To keep things more manageable, this guide will focus on some of the simpler and more impactful math concepts.
If you are brand-new to this work, I highly suggest grabbing a copy of The Poker Math & Preflop Workbook. It contains over 1,000 exercises created to help you learn, practice, and internalize the same calculations you run into during sessions. Each section includes formulas to explain the concept and questions that touch on every subject covered in this guide. Plus, there is an included companion course to help you if you get stuck on a particular chapter.
Remember, you don’t need an IQ of 180 to grasp the math in poker!
Does Poker Strategy Have A Lot Of Math?
Yes, poker involves a significant amount of math. While poker is often considered a game of luck; skill and strategy are also important factors that can significantly influence a player’s success. In order to make informed decisions and improve their chances of winning in the long run, players need to have a solid understanding of the mathematical concepts that underpin the game. So, before you test this out on your favorite us poker sites (some are available to Canadian players as well) keep reading because I’m positive you’ll get a lot from this article.
Poker math involves a range of mathematical concepts, including probability, expected value, pot odds, equity, and more. By understanding these concepts and using them to inform their decision-making process, players can make more informed decisions about whether to bet, call, raise, or fold in any given situation. While it’s not necessary to be a math genius to be a successful poker player, having a good grasp of basic math concepts can go a long way in helping players make the right decisions and improve their results over time.
Then Do Reads Matter In Poker?
Yup, reads also matter. However, most players tend to incorrectly think about approaching poker strategy through either a purely mathematical or purely read-based way. The truth is that combining both allows good reads to inform the assumptions you make about your opponent’s ranges or frequencies, which then updates the variables within the static math formulas.
For instance, say you face a bet on the river holding a bluff-catcher. You can easily calculate the pot odds to see how often you need to be good to justify continuing here (this is the static math formula). If you have a read that your opponent over-bluffs, that informs the variable of how often your bluff-catcher might be. You then can make a better decision using both approaches in tandem.
Poker Mathematics 101
There are tons of calculations you can do when analyzing the game of poker (both at and off the table). However, let’s simplify this down to the poker mathematics that you use the most often.
Any serious poker player understands and uses these concepts regularly.
Additionally, understanding poker math can also help you avoid common mistakes and identify opportunities to exploit your opponent’s weaknesses. So use these mathy poker tips for both new players and seasoned players alike
Poker Strategy Math: Pot Odds
When facing bets and raises in poker, you are always getting odds. This is why you can profitably continue even if you do not rate to have the best hand right at this moment.
Pot odds simply compare the size of the bet you have to call to the size of the pot. They are a mathematical expression of risk and reward that can then be used to make better plays both preflop and postflop.

Whenever you see a statement like “I am getting 2:1 on a call” you are seeing pot odds expressed as a simplified ratio. Essentially, the number left of the colon is the reward, and the number right of the colon is your risk. In the event of getting 2:1 on a call, you are risking 1 unit to win 2 units.
You can then take this ratio and find the equity requirement for continuing profitably. Simply take:
And the number you get is how much equity your hand needs to have in order to make calling break even.
In the event of facing a full-pot bet and getting 2:1 pot odds, you take 1/(1+2) and see that you need at least 33% equity to continue. If your hand’s equity is higher than 33%, you would continue (either by calling or raising).
If your hand’s equity is lower than 33%, you would want to consider future playability and implied odds before you automatically muck your hand.
For quick pot odds calculations you can always use my free pot odds tool here.

Still Not "Getting" Poker Math?
Do you shy away from the math even though you know it would help you play better poker? If yes, this workbook will help you memorize the key formulas, internalize the calculations, and build your intuition to make better decisions at the table.
Get the full-color ebook with 1,500+ questions and a complete answer key today.
The Math Of Combos & Blockers
Combos, combinations, combinatorics. They all mean the same thing. They mean we are looking at ranges from a mathematical perspective and counting the ways our opponent can make certain hands.
Essentially, we are working out how many different ways a specific hand can exist in a given situation.
If you ask a new player how many combos there are of Ace King, they are likely to say “one.” Afterall, AK is just a single hand. But that is not how technical players look at the game. The fact is that there are 16 total combos of Ace King, which includes 4 suited combos and 12 unsuited combos.
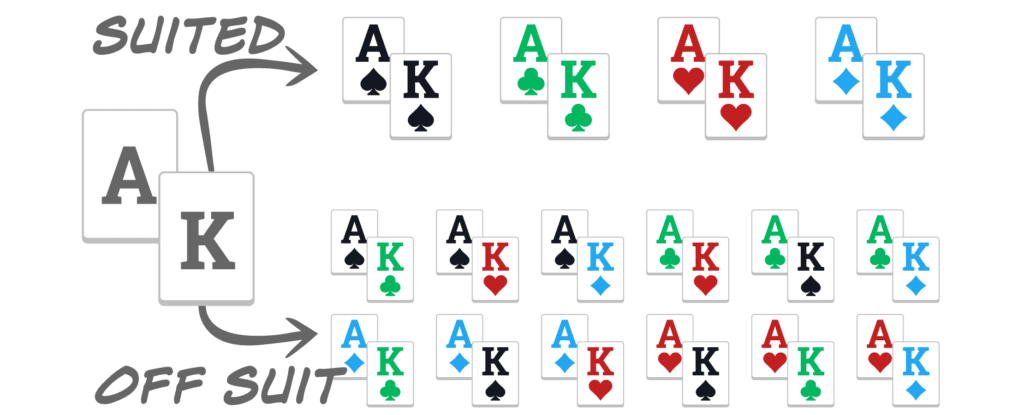
Just remember that there are 6 combos of every pocket pair and 16 of every unpaired hand. The 16 includes both the 12 unsuited and 4 suited versions.
Not too scary, right?
Blockers and combos go hand-in-hand. A blocker is simply a visible card that reduces the combinations of a specific hand. Since there is only one of each rank+suit in the deck, by holding a specific card you make it impossible for your opponents to hold hands that use that specific card.
So if you hold the A♠, it is impossible for your opponent to hold A♠K♠.
If the flop is J♥T♦6♥, the J♥ and T♦ being visible block two possible JTs combos.
Blockers are super important preflop as they can reduce the combos of monster starting hands. When you hold certain hands, say A5s, you reduce the possibility of your opponent holding combos of AA and AK. Note that your A5s does not eliminate ALL possibilities of AA and AK, but minimizing some combinations of strong starting hands can be helpful when finding extra aggression preflop.
When it comes to blockers, there are two rules to remember:
- With pocket pairs, use the 6, 3, 1, 0 rule. This means there are 6 combos with zero blockers, 3 combos if there is a single blocker, 1 combo if there are two blockers, and 0 combos if there are three blockers. So if you hold 77, you block zero combinations of KK, and thus your opponent can have all six combos of KK. If you hold 87s, they can only have three combos of 88. If you hold AA, there is only one possible combo of AA left.
- With unpaired starting hands, multiply together the number of unseen cards. Normally there are 16 combos of all AK (4 unseen Aces * 4 unseen Kings), but if you have ATo, you reduce their AK combos down to 12 (3 unseen Aces * 4 unseen Kings). If you have A♥J♣, they can only have 3 combos of JTs (since the J♣T♣ combo is now impossible).
Poker Strategy Math: Breakeven %
Breakeven percentage (BE%) is the mathematical way of stating when a bet or raise is outright 0EV. If your opponent folds more often than the breakeven %, you make an auto-profit. If they fold less often than the breakeven %, your bluff would take an immediate loss.
That is not to say that all bluffs need to be auto-profitable though. A bluff that fails to be auto-profitable on an earlier street can still be part of a +EV line when factoring in future streets, playability, and edges.
But more on all of that later.
For now, you need to know the formula for this:
Where the risk is the size of your bet or raise, and the reward is the size of the pot you are fighting for. Simply put, the bigger your bet compared to the pot size, the more often your bluff needs to work. The smaller your bet, the less often your bluff needs to work.
Poker Strategy Math: Auto-Profit
A play is auto-profitable when your opponent folds more often than the breakeven percentage (BE%) we just talked about.
So formulaically, that is:
If you bluff for $600 into $600, your BE% comes out to 50%. If you assume your opponent would fold 75% of the time against your $600 bet, then the bluff nets you an auto-profit. Simple.
Auto-profit opportunities are everywhere and they are the focal point for aggressive players. By comparing the BE% to an estimation of how often your opponent will fold to a bluff, you can find extra +EV plays in each session.
This is not to say that we can only bluff if it is auto-profitable since there are other factors at play as well. Rather, by diligently looking for auto-profit spots we can find ways to increase our bluffing frequency in a way that is backed by math.
For more advanced examples of this concept, and many others, be sure to check out my new Advanced Poker Workbook and bridge the gap between GTO and exploitative applications!
Poker Strategy Math: Expected Value (EV)
EV, short for expected value, is the foundational mathematical concept in poker. It is also the primary focus of anyone who is profitable in this game over years and decades.
Our goal is to make as many +EV decisions as possible, and the more +EV the better. In short, a play that is +EV is expected to net us money over the long term while plays that are -EV are going to cost us money over the long term.
The main reason why we focus on EV and the long term is that a single outcome does not tell the whole story.
If you flip a coin, you know it will come up heads 50% of the time and tails 50% of the time. But if you flip the coin once, it will only be one of those. It would be silly to flip a coin once, have it come up heads, and walk away thinking that the result of flipping that same coin daily for the next year will be heads 100% of the time and tails 0% of the time.
Yet, many unstudied players approach poker math in that exact way.
Taking a long term view of your decisions is a better idea. To do this with EV, you can use the simplified EV formula:
This may seem scary at first glance, but with some practice, it gets easier. Eventually, you will frame all of your poker decisions through the lens of EV and have a way to calculate and prove them. For now, though, start by understanding each variable in the formula:
● %W = how often you expect to win this pot
● %L = how often you expect to lose this pot
● $W = when you win, how much you expect to win
● $L = when you do lose, how much you expect to lose
Essentially, the upside of your decision minus the downside of your decision.
There are two key things to keep in mind with this formula:
- %W + %L = 100%. If you expect to win a pot 20% of the time, then inherently you will lose the other 80% of the time. If you know either %W or %L, you can easily find the other.
- A low W% does not automatically mean that a play will be -EV. So long as the $W is a magnitude larger than $L, the play could still be +EV.
If you calculate the EV of a play to be +$27 it means that every time you make that play you expect to make $27. Of course, you can make a +EV play and still lose the pot though. Unless %L is 0%, you will not win that pot every time.
But this is also why we use a bankroll. We need to be comfortable and able to risk money to make +EV plays even if some percentage of the time we will lose the pot.
For additional examples with EV, including a complex EV breakdown, check out my extended EV guide.
Free Poker Math Apps
Want to save yourself hundreds of hours of manually doing long-form poker math and instead use some simple apps?
Grab my free poker spreadsheet pack and get 17 Excel sheets that you can use for everything from calculating EV to runouts to River bet vs. check analysis. The pack is a name-your-own-price product, and yes, you can pay $0 to get them totally for free.

Don’t use Excel? No problem! You can always upload them to Google Drive and open with the Sheets app to use them.

